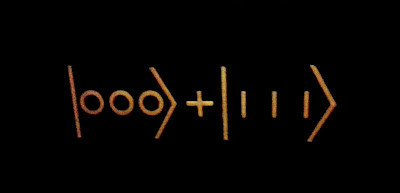Dork Scratchings

Theorem of the week: The Hairy Ball Theorem This says you can't comb a hairy ball without introducing discontinuities such as partings or whorls, unless there's a bald spot.  (There's a more mathematical statement below under the heading "theorem".)  The proof is from An Extremely Short Proof of the Hairy Ball Theorem, by P McGrath , but I've put it into my own words, completely removed all maths notation, and added pictures to make it as accessible as possible.  In addition to being extremely short, it's extremely elegant, and somewhat reminiscent of the Ham Sandwich Theorem . Theorem It is not possible to impose a continuous vector field onto a sphere, such that the vectors are all tangential to the surface, unless the field is zero somewhere Proof Let's assume the sphere does have a continuous, tangential, everywhere non-zero vector field, and attempt to derive a contradiction. Draw a small circle around a point p.  Do one lap around

It may not be entirely obvious from the photograph (which I took at night, while hashing ) but this is a wrought iron gate. It opens on to a front garden on Maid's Causeway, Cambridge.  Why?  I don't know.  Has an important physicist lived there?  There's no Blue Plaque , so maybe it's just an enthusiast, like me! What does it mean?  I don't know for certain, but I suspect it is a reference to something similar to the GHSZ variant of the Bell Inequality Test .  The results of this test demonstrate that there are no hidden variables in quantum mechanics.  I say "similar" because, in GHSZ instead of 0 and 1 the spins $\downarrow$ and $\uparrow$ are used, and there's a minus instead of a plus. If you know anything more, please tell me!

Top left box evolves into a superposition of the other two When introduced to quantum physics the first example we encounter is usually that of a single particle.  We are shown that experiment demonstrates a particle left alone evolves into a superposition of states.  These states may be position states or they may be momentum states, or if the particle has spin it may be a superposition of spin states.  It doesn't matter, the point is that fundamental particles can be in a superposition of states.  That's because they are small, we are told, so you wouldn't expect them to behave like big things do, we are told. The next thing we are shown is how the Schroedinger equation governs the evolution of this superposition.  The particle is not usually in every state equally, it is more in one position state (or momentum state, or spin state) than it is in another.  The distribution over these so-called basis states evolves with time and the Schroedinger equa

Is Our Universe Finite? A while ago I drew the pictures above to try to understand current ideas about the size of the universe. The diagrams are based on some pictures I saw in the book " Our Mathematical Universe ". The diagrams show two dimensional slices of four dimensional spacetime. The blue stuff is "inflationary material" which expands at an enormous rate. The current theory of inflation states that universes like ours form as bubbles in the inflationary material as some of the inflationary material changes phase and "evaporates" out as non-inflationary material. An important point is that the sides of this bubble are moving away from each other way too fast for anything - even light - to travel from one side to the other.  The 1st diagram illustrates the point that in this model there is room for more, far more, than one universe. The yellow region in the 2nd and 3rd diagrams is what is known as a light cone. The point in the middle